Geometry

Some Simple Geometry applied to propagation
and some thoughts on possible mechanisms for some of the phenomena

First a warning......the geometry I am going to explain is used only to give a feeling for the scale of the propagation problem. It should not be thought that it actually happens this like this. Ionospheric propagation is very complicated, but I believe we can get a grasp of the problem by using some simple calculations. I will use the term "reflection" (and reflection height) but it will normally be in single quotes to indicate that this is not physical (mirror-like) reflection, but rather a gradual bending (refraction).

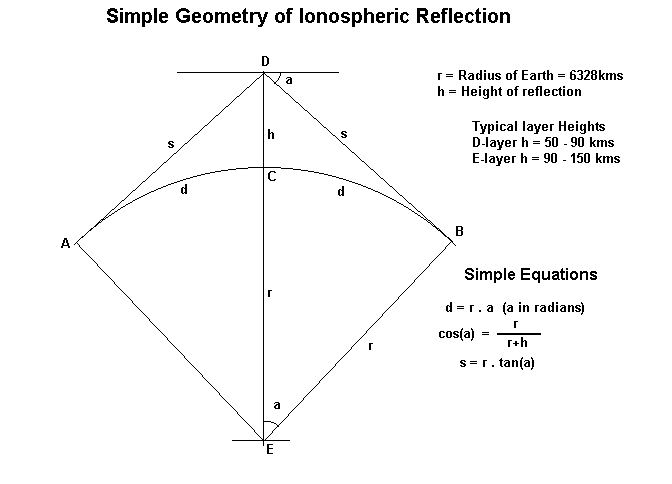
First, consider a radio wave leaving the transmitter site at zero degrees, i.e. horizontally. As the Earth curves away from it, the wave will rise up until it encounters the ionosphere. We will assume that there is a 'mirror' in the sky which reflects the up coming wave in a way like classical optics describes. We can use simple geometry to calculate the distance along the Earth's surface where the wave returns to ground level again. Note two things at this stage, one does not need an aerial with an elevated lobe to transmit skywave, and it is not necessary for this wave to 'bounce', as in classical diagrams, to return to the ionosphere for another 'hop'. At the point where the wave returns to ground level it is travelling parallel to the ground, so it can continue on, up to the ionoshere for a second time. There are practical aspects to this model which I will try to explain later. Sounder results suggest that frequencies around 75 to 150kHz cannot pass though the, daytime, D-layer and return at any detectable strength. This is due to the presence of highly a absorbing region. Also the E-layer does not totally disappear at night as a substantial portion of the ionisation is caused by cosmic rays. It seems generally agreed that night time 'reflection' is from a height comparable with that of the bottom of the E-layer.

The first thing we can do is to calculate the maximum range of a one-hop path in daytime 'reflected' by the D-layer (ref 1.)at an altitude of about 50kms. the equations are given in the diagram below.

If we put the values into the equations with h=50kms, we get the ground distance AB is 1586km, and if we enter the height as 90kms (the bottom of the E-layer for night-time propagation) the distance is 2122km.

Now for the practical points which may modify these values. The ionosphere is not a mirror in the sky, and the return of the wave is not by reflection but rather by refraction. The ray is slowly bent back towards the ground as it enters layers increasing electron density at grazing incidence. This may take many kilometres to achieve and it is feasible that a wave could traverse over a thousand kilometres of the ionoshere before being bent sufficiently to leave the ionoshere and return towards the ground. Those of you who remember clasical physical optics may be a little bemused by this explanation. A wave moving into a medium of higher refractive index is bent into the medium. This must mean that the refractive index of the ionosphere is less than that of air. But hang on a minute air and vacuum has a refractive index of unity, so the refractive index of the ionoshere must be less than one!! and does that not mean that the wave must be travelling faster in the ionosphere than the speed of light in a vacuum, violating Einstein. Well yes and no !! In fact, it depends on the definition of the speed of light. Enistein described it as the speed of carrying information, often now called 'group velocity', what we are saying travels faster in the ionosphere is called the 'phase velocity' or an area of wavefront of uniform phase. Maybe I can make the easier to swallow with an example from all our experience, but explained slightly differently to normal.

Imagine a plane radio wave (phase exactly the same over a plane perpendicular to the direction of travel.) impinging on the directors of a yagi aerial. The wave induces currents in the first director which interact with the wave, and effectively slow the phase front down.

This is further affected by the subsequent directors. It is as if the yagi is slowing down the that portion of the wave close to the boom. The phase front becomes curved and, as the direction of the wave is defined as being perpendicular to the phase front at any point, the wave is directed (focussed) towards the receiving element at the end of the director chain. The aerial gain is achieved because it brings much more of the wavefront into area of influence of the receiving element (dipole) than the dipole would 'see' alone. This form of interaction of the wave with the director chain is achieved by carefully dimensioning the directors to support induced currents with the correct phase. They are reactive at the frequency of the incomming wave. The yagi directors are cut shorter than a resonant half-wave to achieve this. If they were made longer than a resonant halfwave the wave would be bent away from the receiving element. Thus the radio wave is bent without violating Einstein. The ionosphere is a 'cloud' of free electrons and as such is reasonably similar to a metal, and the interaction, between the radio wave and the free electons, changes the direction of the wave distorting the "phase-front".

Another factor which affects the direction of travel of the wavefront leaving the transmit aerial is the dielectric properties of the ground. This has the effect of encouraging the wave to travel slightly slower at the ground surface, bending its direction of travel to follow the curvature of the earth very slightly. The result of this is that the effective take-off angle of a wave from the transmitter can, as measured from the point of contact with the ionosphere, be slightly negative !! Values of up to -10degrees have been calculated. This occurs at both ends of the path and has the effect of lengthening the 'hop' even more. In these conditions, where the wave must hug the ground for a considerable distance before making its way up to the ionoshere, there is more attenuation than for a path which rises above the ground. There are yet more complications. A wave which meets the ionoshere at near grazing incidence will be more easily deflected that a wave impinging at a larger angle of incidence. A high angle wave may well have to penetrate much deeper into the layer before it can be turned groundwards again. The more time it must spend, or distance it must travel, in the ionised layer the weaker it becomes due to absorption.

What does this mean for the two major paths on which we have data, UK (Ipswich)<>CFH (Halifax NS) and CT1DRP (Porto)<>DCF39 (Burg). The surface great circle path from my location to Newport Corner near Halifax Nova Scotia is approximately 4675km, and the path from Brian CT1DRP to Burg, near Magdeburg where DCF39 is located, is 1950km. From the calculations above the one hop night-time path is a maximum of 2122km. So it would be resonable to assume that the main mode of the night-time path is a single hop from the bottom of the E-layer at an altitude of 90km. Under very 'good' conditions the absorption is low (no D-layer) and it may be possible to support a higher incidence angle, 2-hop path. These paths will interfere (in an optical sense) and if approximately the same strength will give higher peaks and very deep cancellations or dips. The daytime path is more difficult to explian, as the geometric path from the bottom of the D-layer at 50 kms is only 1586km. This may be near enough to support a single hop path if we consider the lossy extension of the path by extended diffraction and scattering at ground level. There is rarely rythmical fading in daytime on this path, which indicates to me that one mode predominates, and there is only very weak ground-wave (mountains and dry stony ground in the path). I believe that this is because there is a layer of absorbing medium below the reflection level which severely reduces the strength of the multi-hop signal. Under condtions of very high ionisation such as after a major geomagnetic event, the level of daylight ionisation is such that there is no absorbing medium below the 'reflecting layer'. this leads often to a 10dB emhancement of daytime signals but occasionally also to destructive interference due to multiple paths.

The one hop length of 2122km is obviously too short to support a single hop mode over the Atlantic to CFH Halifax, so it is more likely to be a two-hop mode. This is supported by the observation that the main signal rise at night occurs just about as the solar shadow is reaching the Nova Scotia coast at ground level. The geometry is the same as above so the shadow at 90km altitude is still about 1000km off the coast. This means that three quarters of the bottom of the E-layer is in darkness, and this is just the right conditions for the two 'contact points' of the wave with the 'reflecting layer' to be in darkness. Further there is often a small peak to be seen at the beginning of the main rising front of the signal. The timing suggest (see the plot) that this is the arrival of the two hop signal, but it is often accompanied by a dip. I believe this may be when the single and two hop signals are at about the same strength and there is some destructive interference. Later the single hop signal is swamped by the two-hop signal, but by the middle of the darkness period is often strong enough to produce a destructive interference dip.

There is the question as to why the single-hop signal does not show more clearly. My data was collected at a fairly active period of the solar cycle and I have a lot of evening noise. There has been suggestions from the western side of the Atlantic that they receive an enhanced signal from DCF39 about an hour before sunset. DCF39 is 3dB more powerful than CFH. Add to this the fact that the early evening is the quietest time for man-made noises on the western side, and the explanation gains a little more credence. Further evidence for the posibility of a one hop mode was gained when it was noted that the daytime signal from CFH heard only in the after-effects of a major geomagnetic storm peaked at about 1500z (reported by Mike G3XDV) which is the point where the sun is directly overhead, and thus providing the maximum additional UV flux, in mid-Atlantic. North American East Coast stations have also reported DCF39 peaking around 1500z during daytime enhancements.

I believe that CFH is only heard in daytime because the extra ionisation provided by injection of energetic electrons (often refered to in profession articles as "precipitation" ) from the CME plasma, has driven the reflection layer right down to 50km altitude or even lower. This means that there is little or no absorbing ionisation below the 'reflection level', revealing the signal that is normally well below the noise. At this height one might expect 2 or even 3 hop signals to be stronger, but the extra excursions through any absorbing medium, and the lack of deep cancellations, reduce this possibility. This effect can also be seen on the daytime path between Porto and Burg when there is a major (M-Class or higher) X-ray flare. The radiation from an X-ray flare produces intense ionisation for the period of the flare but the ions recombine rapidly when the flare fades. If a flare occurs on top of a normal daytime signal, the peak signal level is enhanced by up to 10dBs. As the flare fades the level drop well below the normal level and slowly recovers to follow the normal daily.profile. I believe that the intense radiation from the flare, drives the reflection layer down so no absorbing medium remains below the reflection level. The reduced attenuation gives the enhanced level. Then as the UV flux decays the reflection level rises to its normal altitude but there is enough radiation to produce a strongly absorbing layer below this, and hence the dip in signal level is recorded.

Finally there is the phenomena of the early morning quiet period. It had been thought that this would be a good time for DX as the noise is normally at its lowest level all day. However I believe that this quiet period is mainly due to the destruction of ionospheric propagation as the sun rises. If you consider the rising sun at the right of the above diagram. the ground shadow (dawn) is at B. The rays of the sun impinge on the ionoshere at D from underneath !! (2) The solar radiation is somewhat weakened because it has passed through the atmosphere once already. The effect of this is that for a short time the radiation builds up an absorbing layer from the bottom of the D-layer upwards. On an east-west path this would not be too significant as only a small portion of the path would become absorbing. However if the path is aligned close to the shadow, as is the case with predominantly north-south paths the absorption will appear all along the path. I believe this explains the sharp dip at dawn (mid-path) and to a lesser extent at dusk on the plots of DCF39 from Porto. These dips are are also very clear on a long series of plots given to me by Vaino Lehtoranta OH2LX, of DCF39 from Finland. It also explains the difficulty found trying to work Brian from the UK in the early morning.

There are many mechanisms in play affecting the propagation of LF signals, and the relative importance of each one radically affects the end result. The above thoughts are my attempt to see through the 'fog' of the complexity, aided by the data we have collected, and a great many professional observations published in papers buring the last 75 years. Hopefully these attempts to understand the problam at a simple functional level, will aid our exploitation of the 136kHz band.

References

1. Davies K. "Ionosheric Radio" Peter Perigrinus/IEE 1990 Chap. 10

2. Robert R. Brown " More on Atmospheric Ozone and Low-Frequency Propagation " QRX (ARRL) Jan/Feb 2000